Dyadic wavelet transforms are scale samples of wavelet transforms following a geometric sequence of ratio 2. Time is not sampled.
This transform uses dyadic wavelets.
It is implemented by perfect reconstruction filter banks.
The dyadic wavelet transform of f is defined by
It defines a stable complete representation if its Heisenberg boxes cover all of the frequency axis, that is, if there exist A et B such that
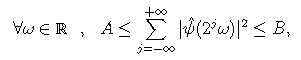
The family of dyadic wavelets is a frame of L2(R).
To build dyadic wavelets, it is sufficient to satisfy the previous condition. To do so, it is possible to proceed as for the construction of orthogonal and biorthogonal wavelet bases, using conjugate mirror or perfect reconstruction filter banks.
The wavelets satisfy then scaling equations and the fast dyadic wavelet transform is implemented using filter banks.
The fast dyadic wavelet transform uses the same filters as for the computation of the fast wavelet transform of a discrete signal, except that no subsampling is performed.